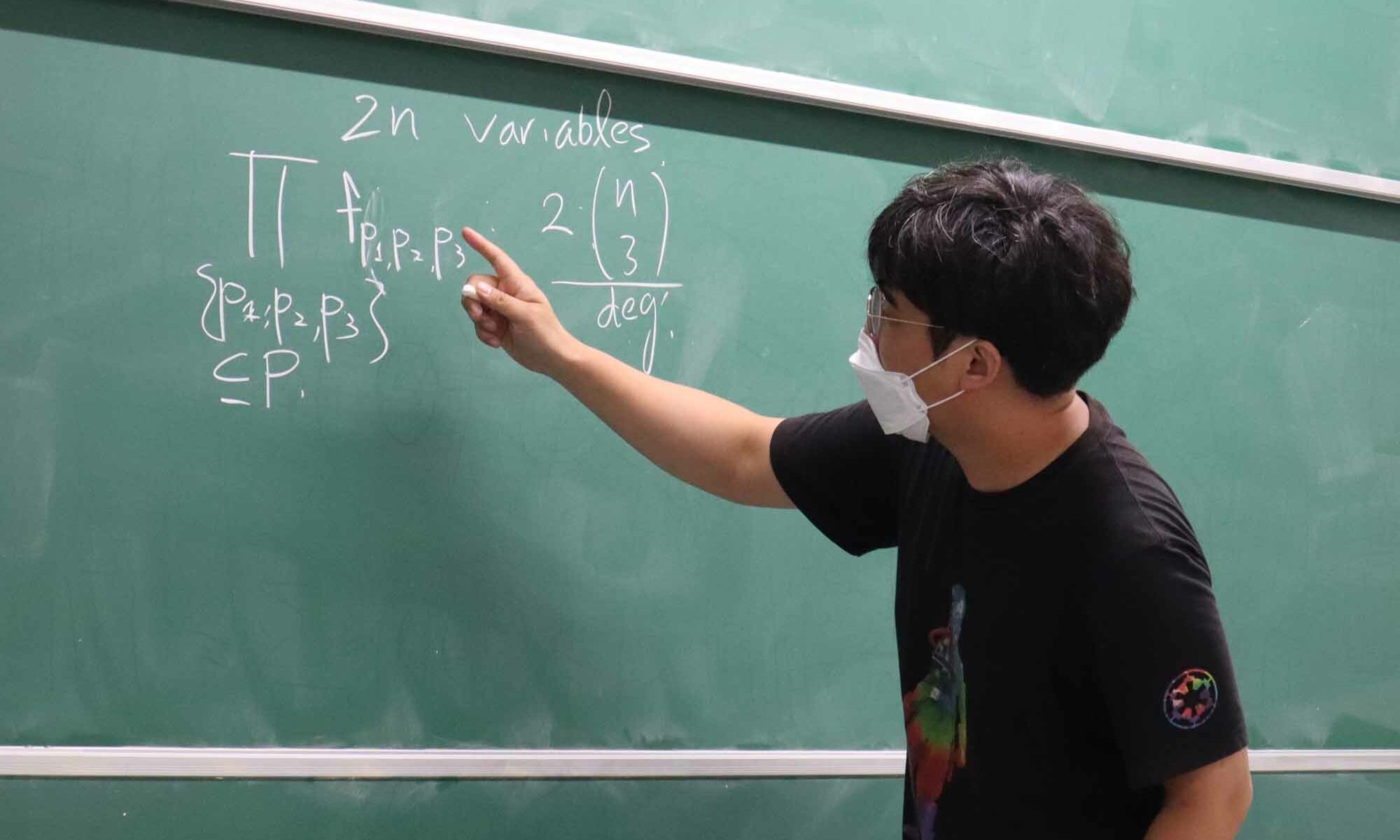The IBS Discrete Mathematics Group welcomes Dr. Seunghun Lee, a new research fellow at the IBS Discrete Mathematics Group starting July 1, 2025. He received his Ph.D. from KAIST in August 2020. He was a Robert Riley visiting assistant professor at Binghamton University in the USA from August 2020 to July 2022, a postdoctoral research fellow at Hebrew University of Jerusalem from August 2022 to January 2024, and a BK21 Research Assistant Professor at KAIST from 2024. Welcome!
Seunghun Lee (이승훈) gave a talk on the existence of hypergraphs embeddable in ℝ^d with large chromatic number at the Discrete Math Seminar
On November 20, 2023, Seunghun Lee (이승훈) from the Hebrew University of Jerusalem gave a talk at the Discrete Math Seminar on the existence of k-uniform hypergraphs embeddable in ℝ^d with large chromatic number. The title of his talk was “On colorings of hypergraphs embeddable in R^d.”
- Due to technical issues, some portions of the videos were not adequately recorded on YouTube.
Seunghun Lee (이승훈), On colorings of hypergraphs embeddable in
Given a hypergraph
Upon my previous presentation, which is based on the joint work with Joseph Briggs and Michael Gene Dobbins (https://www.youtube.com/watch?v=WLY-8smtlGQ), we update what is discovered in the meantime about transversals and colororings of geometric hypergraphs. In particular, we focus on chromatic numbers of
Seunghun Lee (이승훈) gave a talk on order types realized by a point configuration whose extreme points are cocircular at the Discrete Math Seminar
On August 1, 2022, Seunghun Lee (이승훈), who is from the Binghamton University, and will be soon at the Hebrew University of Jerusalem, gave a talk at the Discrete Math Seminar on order types realized by a point configuration whose extreme points are cocircular. The title of his talk was “Inscribable order types“.
Seunghun Lee (이승훈), Inscribable order types
We call an order type inscribable if it is realized by a point configuration where all extreme points are all on a circle. In this talk, we investigate inscribability of order types. We first show that every simple order type with at most 2 interior points is inscribable, and that the number of such order types is
Seunghun Lee (이승훈) gave a talk on the transversal number and the chromatic number of hypergraphs consisting of facets of a simplicial sphere at the Discrete Math Seminar
On January 4, 2022, Seunghun Lee (이승훈) from the Binghamton University gave a talk at the Discrete Math Seminar on the transversal number and the chromatic number of hypergraphs whose edges are facets of a simplicial sphere. The title of his talk was “Transversals and colorings of simplicial spheres“.
Seunghun Lee (이승훈), Transversals and colorings of simplicial spheres
Motivated from the surrounding property of a point set in
Seunghun Lee (이승훈) gave a talk on the complexes of subgraphs having no large matching at the Discrete Math Seminar
On April 28, 2020, Seunghun Lee (이승훈) from KAIST presented a talk on the topological property of the non-matching complex, that is a simplicial complex consisting of subgraphs on the same vertex set having no matching of size k and its application to the rainbow matching problem of graphs. The title of his talk is “Leray numbers of complexes of graphs with bounded matching number“.
Seunghun Lee (이승훈), Leray numbers of complexes of graphs with bounded matching number
Given a graph
This is a joint work with Andreas Holmsen.