On April 4, 2023, István Tomon from Umeå universitet, Sweden gave a talk at the Discrete Math Seminar on the intersection graph of axis-parallel boxes at the Discrete Math Seminar. The title of his talk was “Configurations of boxes.”
Configurations of axis-parallel boxes in are extensively studied in combinatorial geometry. Despite their perceived simplicity, there are many problems involving their structure that are not well understood. I will talk about a construction that shows that their structure might be more complicated than people conjectured.
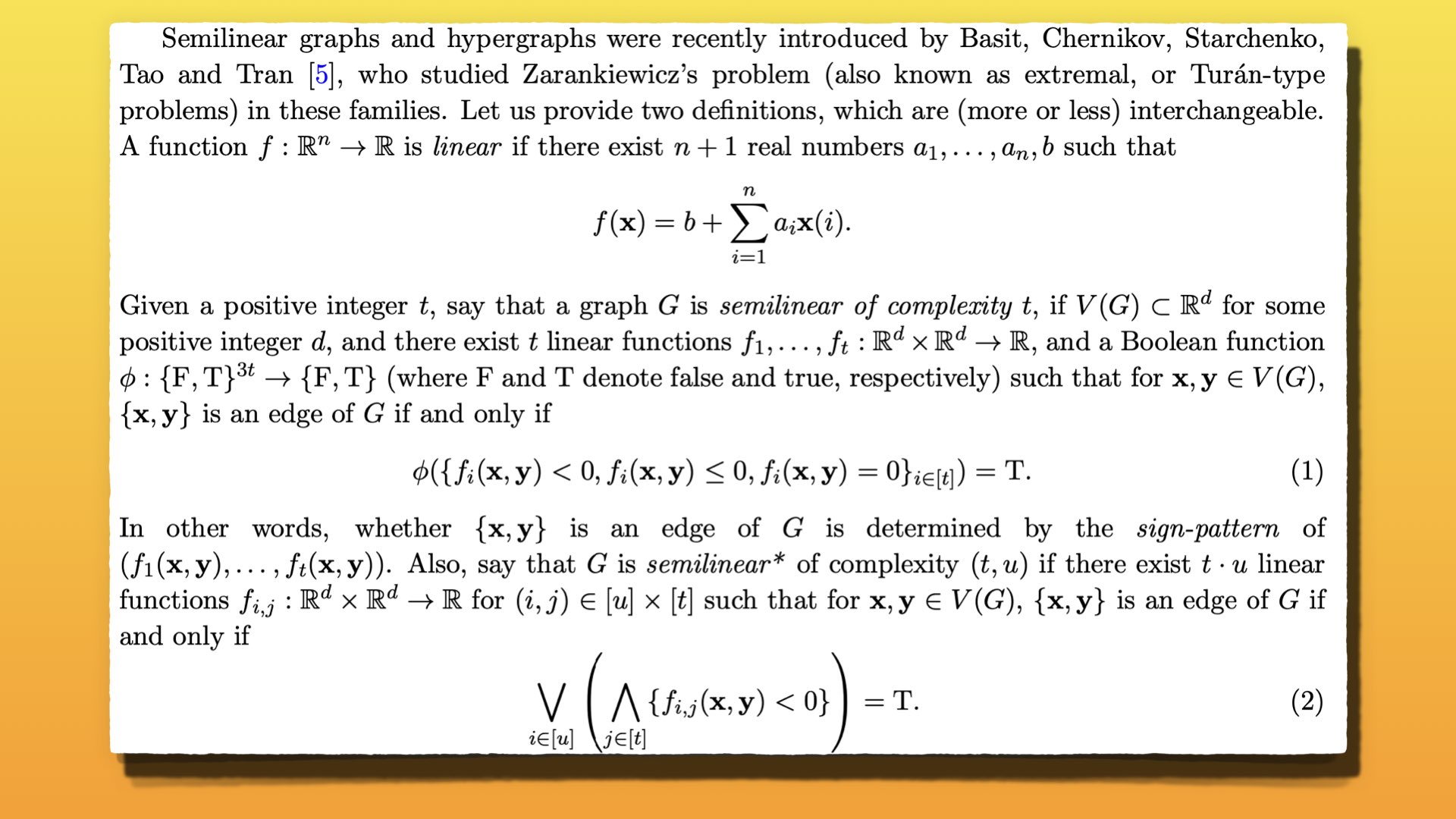
On April 14, 2021, István Tomon from the ETH Zurich gave an online talk at the Virtual Discrete Math Colloquium on the size of the maximum clique, the size of the maximum independent set, and the chromatic number of a semilinear graph of bounded complexity. The title of his talk was “Ramsey properties of semilinear graphs“.
A graph is semilinear of bounded complexity if the vertices of are elements of , and the edges of are defined by the sign patterns of linear functions, where and are constants. In this talk, I will present several results about the symmetric and asymmetric Ramsey properties of semilinear graphs. Some interesting instances of such graphs are intersection graphs of boxes, interval overlap graphs, and shift graphs, so our results extend several well known theorems about the Ramsey and coloring properties of these geometrically defined graphs.
