기초과학연구원 이산수학그룹
On September 17, 2020, Luke Postle from the University of Waterloo gave an online talk about his recent (another) breakthrough towards Hadwiger’s conjecture. The title of his talk was “Further progress towards Hadwiger’s conjecture“.
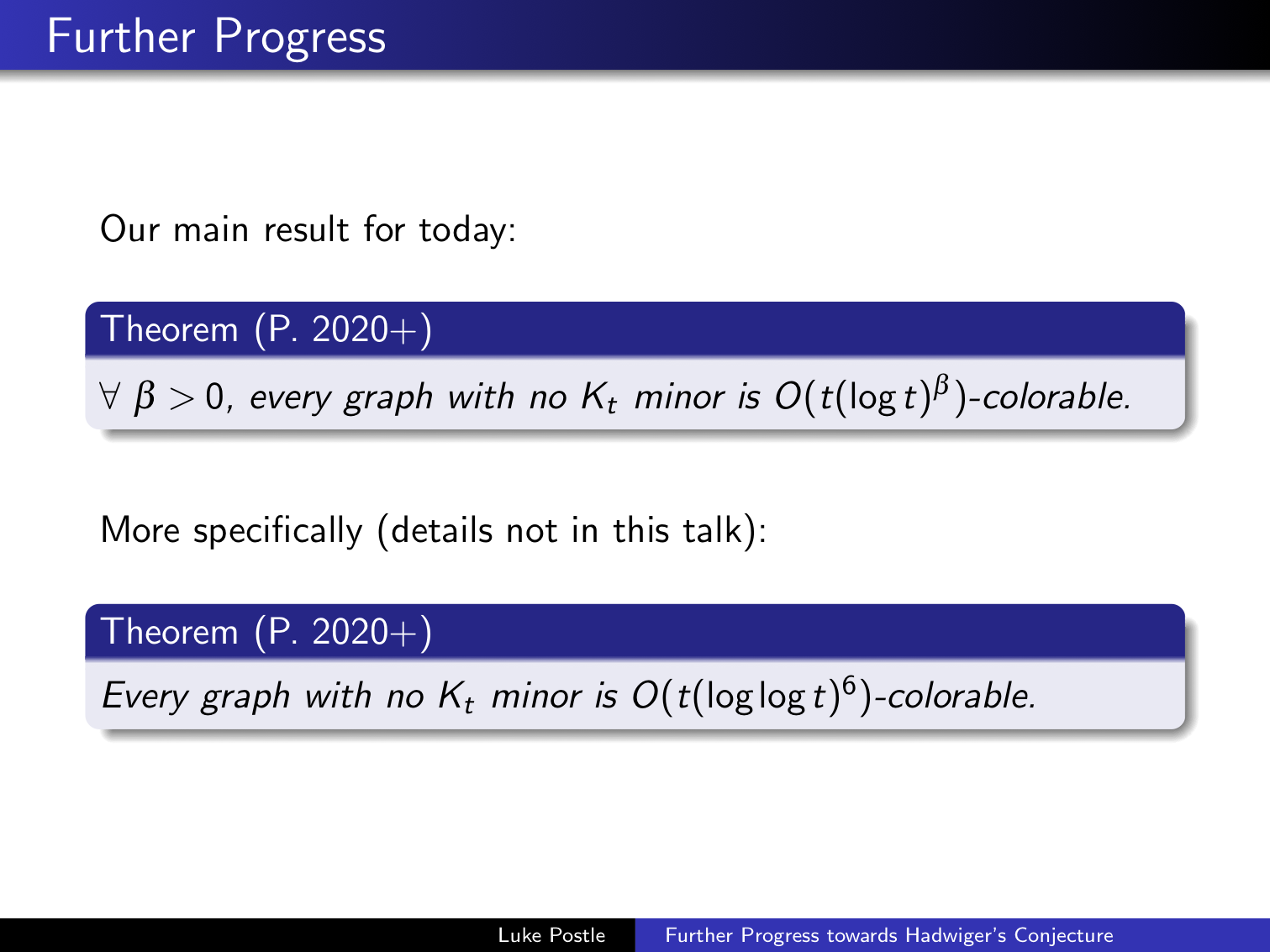
In 1943, Hadwiger conjectured that every graph with no $K_t$ minor is $(t-1)$-colorable for every $t\ge 1$. In the 1980s, Kostochka and Thomason independently proved that every graph with no $K_t$ minor has average degree $O(t\sqrt{\log t})$ and hence is $O(t\sqrt{\log t})$-colorable. Recently, Norin, Song and I showed that every graph with no $K_t$ minor is $O(t(\log t)^{\beta})$-colorable for every $\beta > 1/4$, making the first improvement on the order of magnitude of the $O(t\sqrt{\log t})$ bound. Here we show that every graph with no $K_t$ minor is $O(t (\log t)^{\beta})$-colorable for every $\beta > 0$; more specifically, they are $O(t (\log \log t)^{6})$-colorable.