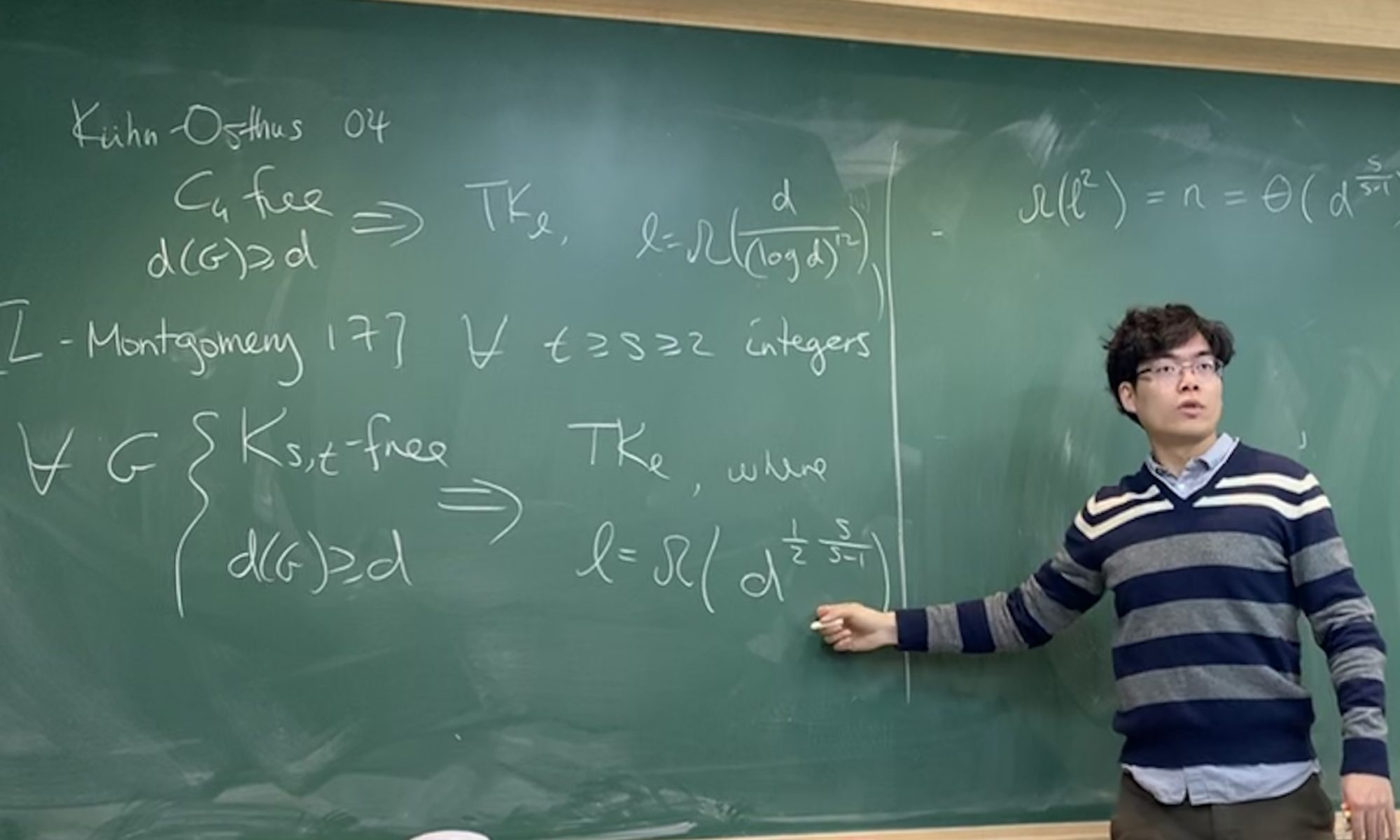This lecture series covers several different techniques on embedding paths/trees/cycles in (pseudo)random graphs/expanders as (induced) subgraphs.
Hong Liu (刘鸿), Cycles and trees in graphs (6/8)
This lecture series covers several different techniques on embedding paths/trees/cycles in (pseudo)random graphs/expanders as (induced) subgraphs.
Hong Liu (刘鸿), Cycles and trees in graphs (5/8)
This lecture series covers several different techniques on embedding paths/trees/cycles in (pseudo)random graphs/expanders as (induced) subgraphs.
Hong Liu (刘鸿), Cycles and trees in graphs (4/8)
This lecture series covers several different techniques on embedding paths/trees/cycles in (pseudo)random graphs/expanders as (induced) subgraphs.
Hong Liu (刘鸿), Cycles and trees in graphs (3/8)
This lecture series covers several different techniques on embedding paths/trees/cycles in (pseudo)random graphs/expanders as (induced) subgraphs.
Hong Liu (刘鸿), Cycles and trees in graphs (2/8)
This lecture series covers several different techniques on embedding paths/trees/cycles in (pseudo)random graphs/expanders as (induced) subgraphs.
Hong Liu (刘鸿), Cycles and trees in graphs (1/8)
This lecture series covers several different techniques on embedding paths/trees/cycles in (pseudo)random graphs/expanders as (induced) subgraphs.
Hong Liu gave a talk describing approximate structures of an n-vertex m-edge graph minimizing the number of cliques of size k at the Discrete Math Seminar
On May 26, 2020, Hong Liu from University of Warwick presented a talk on his recent work describing approximate structures of an n-vertex m-edge graph minimizing the number of cliques of size k. The title of his talk was “Asymptotic Structure for the Clique Density Theorem”.
Hong Liu (刘鸿), Asymptotic Structure for the Clique Density Theorem
The famous Erdős-Rademacher problem asks for the smallest number of r-cliques in a graph with the given number of vertices and edges. Despite decades of active attempts, the asymptotic value of this extremal function for all r was determined only recently, by Reiher [Annals of Mathematics, 184 (2016) 683-707]. Here we describe the asymptotic structure of all almost extremal graphs. This task for r=3 was previously accomplished by Pikhurko and Razborov [Combinatorics, Probability and Computing, 26 (2017) 138–160].
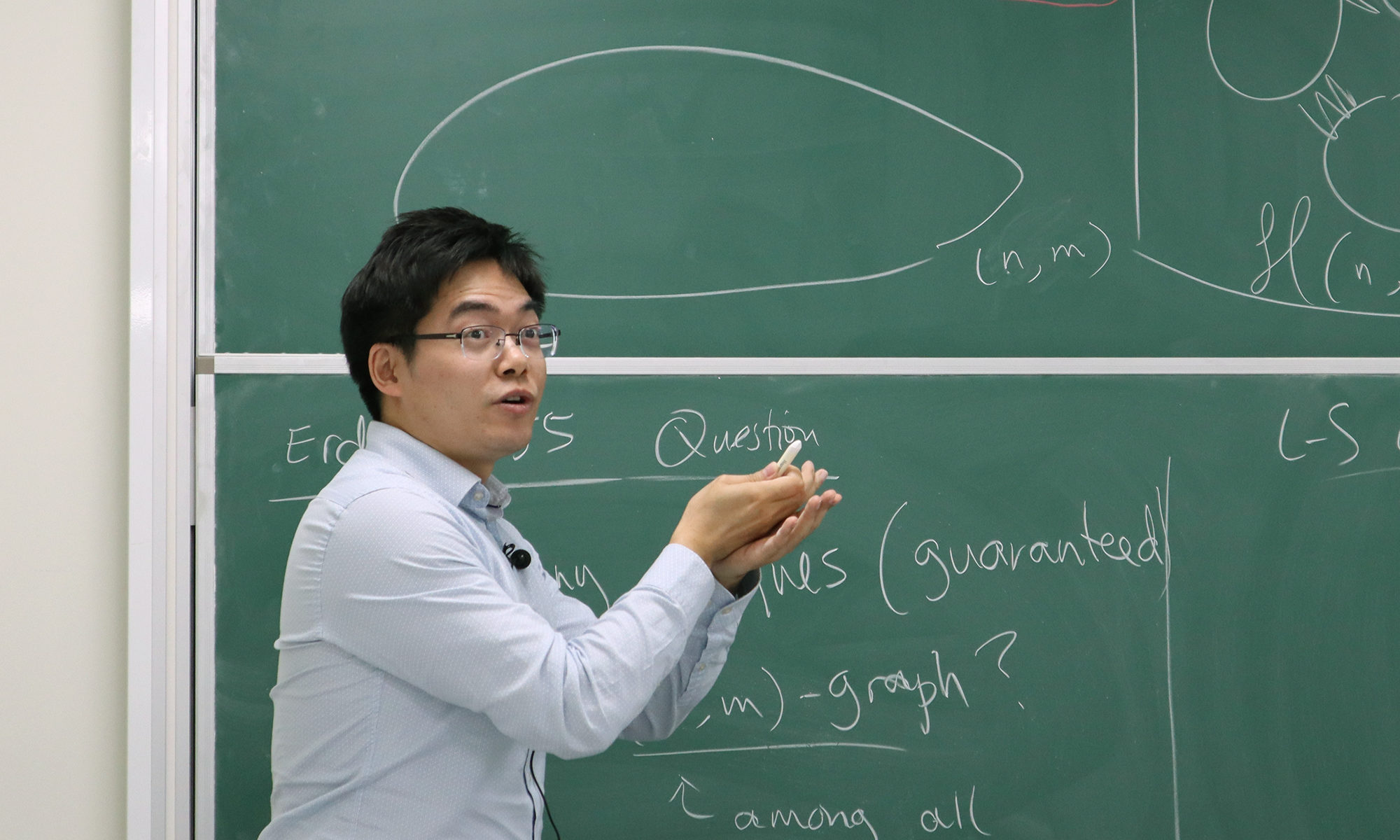
Hong Liu gave a talk on the conjecture of Mader about the topological minors in C4-free graphs at the discrete math seminar
On December 12, 2019, Hong Liu from University of Warwick gave a talk on the resolution of the conjecture of Mader on the existence of a complete topological minor under the condition of average degree for C4-free graphs at the discrete math seminar held at KAIST. The title of his talk was “A proof of Mader’s conjecture on large clique subdivisions in