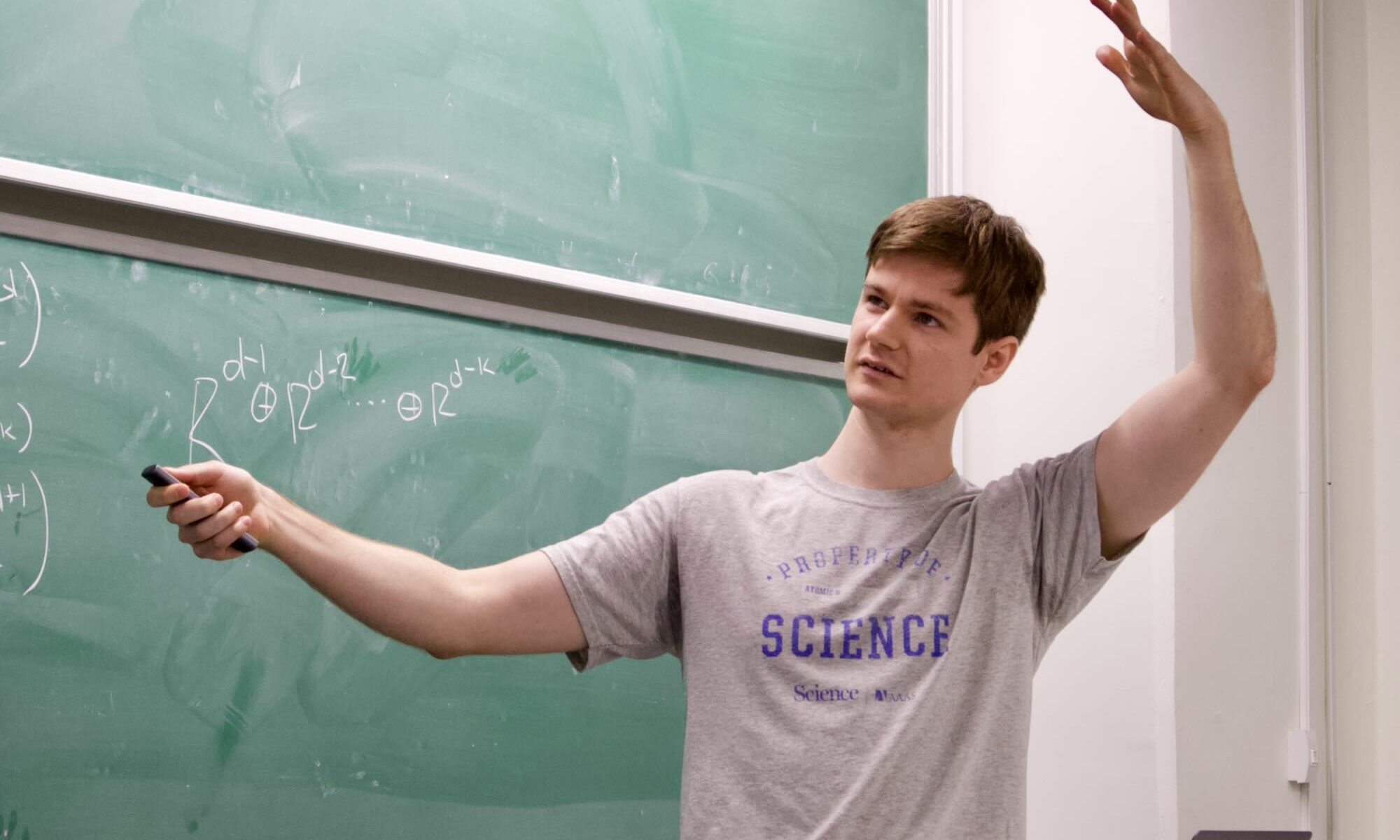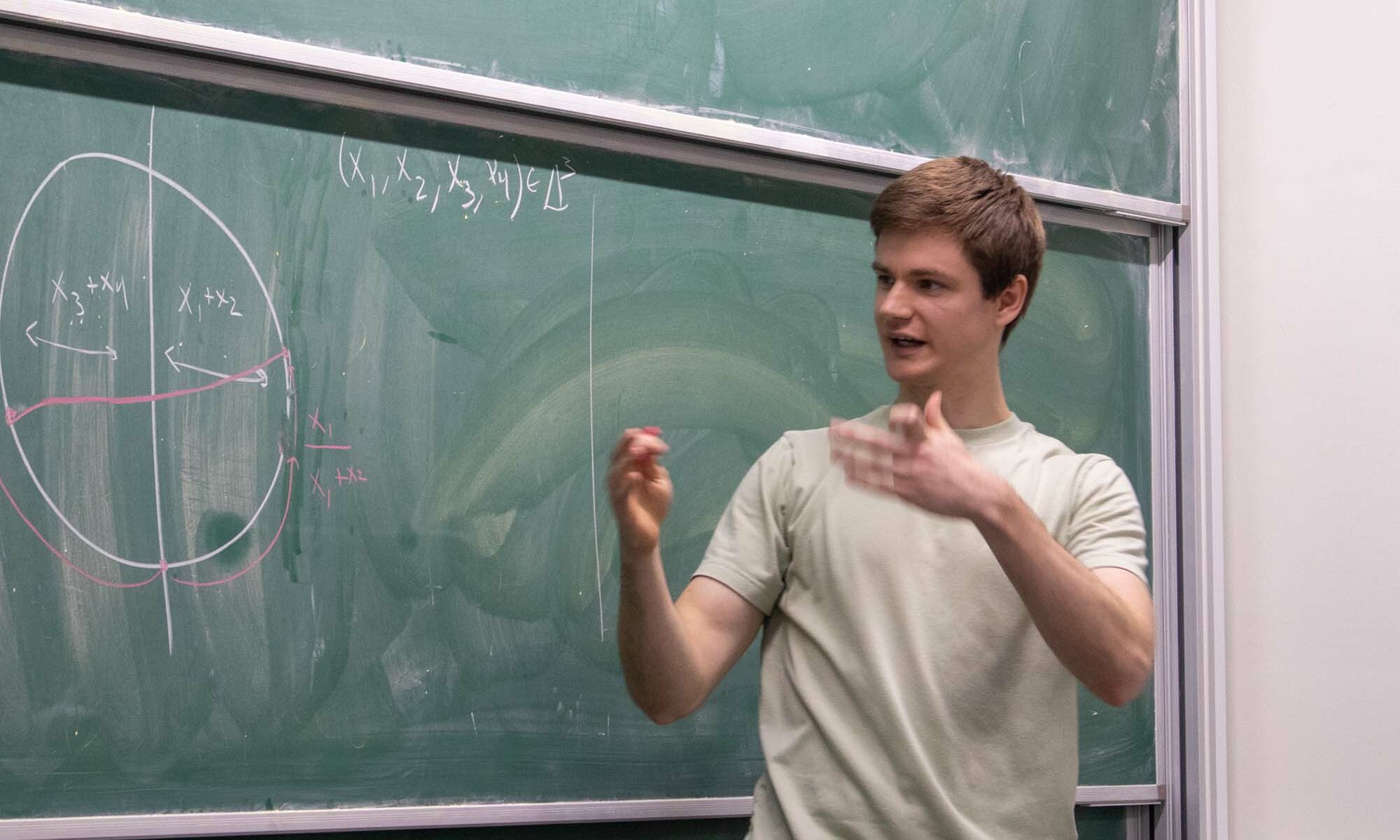기초과학연구원 이산수학그룹
On January 2, 2024, Daniel McGinnis from Iowa State University gave a talk at the Discrete Math Seminar on applications of the KKM theorem to various problems in discrete geometry. The title of his talk is “Applications of the KKM theorem to problems in discrete geometry“.
We present the KKM theorem and a recent proof method utilizing it that has proven to be very useful for problems in discrete geometry. For example, the method was used to show that for a planar family of convex sets with the property that every three sets are pierced by a line, there are three lines whose union intersects each set in the family. This was previously a long-unsolved problem posed by Eckhoff. We go over a couple of examples demonstrating the method and propose a potential future research direction to push the method even further.